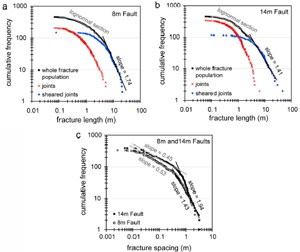
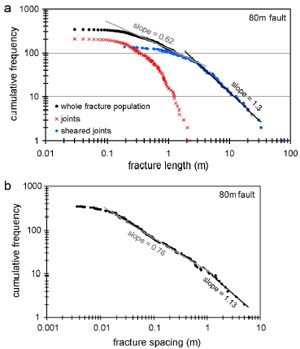
As discussed in the section 'Fault Damage Zones,' de Joussineau and Aydin (2005, 2007) characterized the inner and outer damage zones of strike-slip faults with larger than 5 meter slip in Aztec Sandstone exposed in Valley of Fire State Park, Nevada. Parallel scanlines are measured across three damage zone of increasing apparent maximum slip magnitudes of 8, 14, and 80 meters.

The cumulative fracture-length and fracture-spacing distributions for the 8 m and 14 m faults are plotted in Figure 1. The fracture-length distributions (Figures 1(a) and (b)) are similar for the two faults with log-normal distributions for the smaller fracture lengths and fractal (power law) distributions for the larger fracture lengths. The shift in the fracture-length distributions is particularly easy to observe for fracture lengths corresponding to the longest joints (5.4 m and 3.95 m, respectively, for Figures 1(a) and (b)). The log-normal part of the distributions corresponds to joints and sheared joints while the steep fractal part of the distributions is comprised of sheared joints or small faults only. All of the relatively long fractures are sheared, indicating that shearing results in increased fracture length and (or) that long fractures are prone to slip more than shorter fractures. The fracture-spacing distributions are also similar for the two faults (Figure 1(c)) and are multifractal with two straight segments (bi-fractal).

Figure 1. (a and b) Plots showing cumulative fracture-length frequency distributions for the damage zone of the 8 m fault (a) and 14 m fault (b), based on eight parallel scanline surveys for each fault. Distributions are log-normal for short fractures and fractal for longer fractures. Slopes of the fractal segments are provided. (c) Plots showing cumulative fracture-spacing frequency distributions for the damage zone of the 8 m Fault and the 14 m fault based on the same surveys as (a) and (b). Distributions are bi-fractal. Slopes of the fractal segments are provided. From de Joussineau and Aydin (2005).

The cumulative fracture-length and fracture-spacing distributions for the 80 m fault are plotted in Figure 2. The two distributions are multifractal with two straight segments (bi-fractal). As was the case for the 8 m fault and the 14 m fault, the shift in the fracture-length distribution occurs for a fracture length approximately corresponding to the longest joints (3.04 m, Figure 2(a)). The fractal segment with the smaller slope corresponds to small fracture lengths, defined by joints and slightly sheared joints, while the steep fractal segment corresponds to large fracture lengths, defined solely by long sheared joints or small faults.

Figure 2. Plots showing (a) the cumulative fracture-length frequency distribution and (b) the cumulative fracture-spacing frequency distribution for the damage zone of the 80 m fault. Distributions are bi-fractal and slopes of the segments increase for large scales, as marked. From de Joussineau and Aydin (2005).